Many universities require students to take mathematics courses at some time during their undergraduate career. These mathematics courses require you to be able to understand theories and apply them. Math courses, exams, and textbooks can vary quite differently from other university courses, and the following guides have been created to help you navigate these differences.
Image © studyright.net
Meet with a peer tutor for a 25-minute tutoring session to help you with study skills and questions about your course work:
Book a Real-Time Online Tutoring Session
External Resources
Using Math Textbooks
Math textbooks are unique because they use math language to communicate. To be successful in a math course, students need to be ready to learn this language and how to use it to communicate effectively.
Math textbooks differ from other textbooks in three ways:
- Math texts present information in a very compact form.
For example, a graph of a function contains a great deal of information, such as function behaviour, x and y intercepts, maximum and minimum value of the function, and much more, depending on the function. - Math textbooks provide problem solving techniques and approaches.
The goal is to help students develop problem solving and critical thinking skills through examples, formulas and a variety of written and visual aides. - Every chapter in a math textbook contains purposefully designed practice exercises.
Because learning math requires understanding underlying concepts and applying problem solving skills, practice exercises help the learner develop understanding as well as problem solving techniques/skills and thinking.
Tips and Techniques
How to us the Tips
Preview the text before the class by skimming for broad ideas and familiarize yourself with the vocabulary. This helps you get ready to listen to the teacher’s explanations.
|
Watch for italics, colour, boxes, and other methods that the author uses to catch your attention. |
|
Use the index when you want to find the meaning of a word that you don’t understand. |
|
Always take notes as your read. Reading math textbooks requires that you have a pencil and paper (and probably a calculator) to simplify the information by noting down only the key information, definitions, and formulas that you need to memorize. Try to make these notes your own by using your own words, or examples from your own life. |
| Spend extra time reading and understanding diagrams and example problems to have a good idea of the type of problems that you will solve and the basic idea of the approach to solving them. Try some examples by yourself to examine your understanding. |
|
Since practice is important, you should try one or more problems from each section and make a note of their differences. |
|
Write down the steps to solve each problem so you can follow the same steps when you work with similar problems. |
|
Most math texts have chapter tests at the end of each chapter. Take at least some the test problems to test yourself before the exam. |
External Resources
Math tests will assess your understanding of the concepts and your skill at applying these concepts. There may be multiple choice, problem solving, and calculation questions that will ask you to solve, evaluate, and calculate.
Tips and Techniques
|
1. Briefly skim through the whole test before you begin to find out what are the easiest and hardest parts so you can plan your time. |
|
2. Identify which sections count for the most points. |
|
3. Read the directions very carefully so that you follow the requested steps completely. |
|
4. Being well prepared for the test is the best way to reduce anxiety. |
|
5. Continually reviewing the class material over weeks or days is the key to success. (Don’t wait till the night before!) |
|
6. Do the simple questions first to help you build up your confidence for the harder questions. |
|
7. Don’t worry about how fast the other classmates finish their test. When considering exam timing, just concentrate on your own test. |
Example sequence for taking a math test
- Calculations - they can give you the most confidence because you can check your answers and make sure that they are right.
- Problem Solving - because these questions usually count for the most marks on exams, a good strategy is to spend more time on this section.
- Multiple Choice - these questions are usually worth the least marks so spend less time on each.
Please note that this is just an example and you should rearrange this sequence based on your own preference.
Tips for calculations questions
- Step by step. Do not skip any step(s) when solving these questions. Write down all the steps you took and show all your work.
- Make a clear layout so that it will be easier to go back and check your work if you run into problems along the way solving the question.
- Check or redo calculations to make sure that your answer is right (if there is enough time). You can either do this right away or return to the calculation after finishing the rest of the exam.
Strategies for Problem Solving
- Extract relevant information/numbers from the problem, list them on your paper, and determine what they are.
e.g. principle = 200,000 dollars, year = 5 years, interest = 2.3% - Understand what the question is asking you by highlighting, circling, or underlying key words.
e.g. what is the future value? - Identify the formula that you need to use.
e.g. y = mx + b
Example
- Extract numbers from the problem, list them and determine what they are.
(e.g. principle = 200,000 dollar, year = 5 years, interest = 2.3% annually) - Understand what the question is asking by highlighting, underlining, or circling key words.
(e.g. what is the future value) - Create Cost and Revenue formulas:
Equations: X = unit of sale (production) Cost formula: C(X) = variable cost × X + fixed cost Revenue formula: R(X) = selling cost × X - With the Cost formula, find the following:
- What is the Y-Intercept? (O, Y)
- What is the X-Intercept? (X1, 0), (X2, 0)
- What is the Max/Min?
- Where does the graph start? (X, Y)
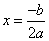
- With the Revenue formula, find the following:
- What is the Y-Intercept? (O, Y)
- What is the X-Intercept? (X1, 0), (X2, 0)
- What is the Max/Min?
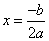
- Where does the graph start? (X, Y) - Find your Break-Even:
(note: at Break-Even your profit is 0)Profit = revenue - cost
(X1, Y1), (X2, Y2)
- Now you should have enough information to plot your graph.
Remember to label every single point you have found above, and also LOSS and PROFIT!